How to Find the Height of a Triangle with Base and Area
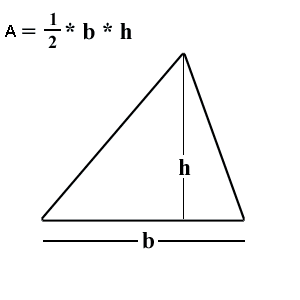
Let us consider a triangle with three vertices A, B and C. If you draw a perpendicular line starting from the vertex A and ending at the point where it meets the side of the triangle which is opposite to vertex A, BC in this case, then that line will be the height of the triangle. Line segment BC is the base of the triangle in this case.
Here is how you will be able to find the height of a triangle if you know the area and base length of that particular triangle.
Instructions
-
1
We will find the height of the triangle ABC using the simple mathematical formula which says that the area of a triangle (A) is one half of the product of base length (b) and height (h) of that triangle.
Area of triangle (A) = ½ × Length of the base (b) × Height of the triangle (h) -
2
Let us assume that area of the triangle (A) is 35cm² and length of the base (b) is 7cm. This means
A = 35cm²
b = 7cm -
3
Read the formula written in the first step once again. You will see that it depends on three variables A, b and h. We already have values for A and b so the only unknown variable left is h, the height of the triangle. If we slightly modify the formula, we can calculate the exact height of the triangle.
-
4
Area of triangle (A) = ½ × Length of the base (b) × Height of the triangle (h)
A = ½ × b × h
2 × A = b × h
(2 × A) / b = h
h = (2A)/b -
5
Simply put in values of A and b to calculate the height of the triangle.
h = (2 × 35) / 7
h = 70 / 7
h = 10cm -
6
You may want to check the value of height that you just calculated to see if it is correct or not. Simply substitute the value of h in the original formula and calculate the area of the triangle.
Area of triangle (A) = ½ × Length of the base (b) × Height of the triangle (h)
A = ½ × 7 × 10
A = 7 × 5
A = 35cm²
Since the value of area is the same as was originally given, this means that the value you calculated for the height of the triangle is correct.